Each chapter includes step-by-step solutions to all exercises and examples as per the new book prescribed by SEBA (SCERT Assam) for Class 7.
Free PDF of SCERT Assam Class 7 Mathematics Chapter 1 – Integers includes all the exercise solutions in detail as per the latest SEBA New Book guidelines. These comprehensive solutions are meticulously prepared by Ospin Academy to help students understand the chapter better and achieve good marks in their school exams.
Next: Explore SEBA Class 7 Maths Chapter 2 Fractions and Decimals Solutions!
Enhance your understanding with step-by-step solutions for all exercises. Click here to access Chapter 2 solutions now!
Class 7 Maths (English Medium) PDF Solutions 2025-26 | SCERT Assam
Get Class 7 SEBA Mathematics Solutions PDF for SCERT Assam, covering all chapters with detailed explanations. Each chapter includes MCQs for better practice, along with solved exercises and study notes to help you prepare effectively. Perfect for exam revision and concept clarity!
Exercise – 1.1 |
|---|
1. How many integers are there in between 5 and (-13)?
Ans: Numbers of integers in between 5 and (-13) are: -12, -11, -10, -9, -8, -7, -6, -5, -4, -3, -2, -1, 0, 1, 2, 3, 4 = 17.
2. Write greatest and smallest integers in between 12 and (-13)?
Ans: The greatest and smallest integers in between are 12 and -12 respectively.
3. Plot the following integers on number line.
-6, 4, -10, 5, -1
Ans:
4. Write 5 negative integers which are greater than -15.
Ans: 5 negative integers greater than -15 are: -14, -13, -12, -11, -10.
5. Mention if true or false-
(i) Positive integers are called as Natural Number.
Ans: True.
(ii) All the integers are whole number.
Ans: False.
(iii) Number line is extended to infinity on the both sides of ‘0’.
Ans: True.
(iv) ‘0’ and negative integers form the collection of whole number.
Ans: False.
(v) If a + b = 0, then one of them is additive inverse of other and vice versa.
Ans: True.
6. Write a pair of integers-
(i) Whose sum is -3
Ans: 7 and -10.
(ii) Whose difference is -5
Ans: = 10 – (-5)
= -10 + 5 = -5
(iii) Whose sum is 0
Ans: = 100 + (-100)
= 100 – 100 = 0
(iv) Whose difference is 2
Ans: = 9 – 7 = 2
7. Write a pair of negative integers whose subtraction is 6.
Ans: = -11 – (-17)
= -11 + 17
= 6
৪. Find the integers a and b such that-
(i) a + b is positive.
Ans: a + b positive
Let, a = 5, b = 3
∴ a + b = 5 + 3 = 8 positive.
(ii) a ≠ b
Ans: a ≠ b
a = 5, b = -2
a ≠ b
(iii) a – b = 0
Ans: a – b = 0
a = 9 and b = 9
a – b = 9 – 9 = 0
9. Fill in the boxes:
(i) (-15) + (-4) = (-4) + ……।
Ans: (-15) + (-4) = (-4) + -15
(ii) ……. + {(-7) + 8} = {5 + (-7)} + 8
Ans: 8 + {(-7) + 8} = {5 + (-7)} + 8
(iii) (-23) + ……. = -23 = (-23) + …….।
Ans: (-23) + 0 = -23 = (-23) + 0
(iv) (-19) + …… = (-27)
Ans: (-19) + -8 = (-27)
(v) x + 12 = 0 হ’লে x = ……।
Ans: x + 12 = 0 হ’লে x = -12
10. A man moved 14 kilometers towards East from the position A. But another man moved 6 Kilometers towards West from the position A. What is the distance between them?
Ans: A man moved 14 kilometers towards East from the position A. But another man moved 6 kilometers towards West from the position.
Now, distance moved towards east = +14km . And, distance moved towards west = -6 km.
∴ The distance between them the = +14 – (-6) km.
= (14 + 6) km
= 20km.
11. A man has a deposited ₹ 35 and another man has a debt of ₹ 40. How much rich is first man compared to second man?
Ans: The first man compared to the second man, the amount of rich = Rs. = (35 + 40) = Rs. 75.
12. On a certain Tuesday temperature of Guwahati at 5 am in the morning was 25° C. But temperature was in- creased by 8°C at 2 pm in the afternoon and at 10 pm the temperature was decreased by 3°C. On Wednesday at 12 noon again temperature was increased by 5°C. What was the temperature at 12 noon on Wednesday?
Ans: The temperature at 12 noon on Wednesday = 25°C + 8°C – 3°C + 5°C
= 38°C 3°C
= 35°C
13. Anuradha had deposited Rs. 3200 in the bank and next day she had withdrawn Rs. 2,540. How much money left in the account of Anuradha after withdrawal?
Ans: Money left in the account of Anuradha after with- drawal = Rs. (3200-2540)
= Rs. 660.
14. Sum of two numbers is -5. If one of the number is 18 then what is the other number?
Ans: Sum of two numbers is = -5 and one of them = 18.
∴ Other number = -5 – 18 = -23.
15. What should be added with – 23 to get 0?
Ans: The required number = 0 – (-23) = 23.
16. Sum of two integers is -48. If one of the number is -20 then what is the other number?
Ans: Sum of two integers is = -48 and one of them is = -20.
∴ The other number is = -48 + 20 = -28.
17. Evaluate using number line:
(i) (+5) – (+3)
Ans: (+5) – (+3)

(ii) (+6) + (-5)
Ans: (+6) + (-5)

(iii) (-6) – (+5)
Ans: (-6) – (+5)
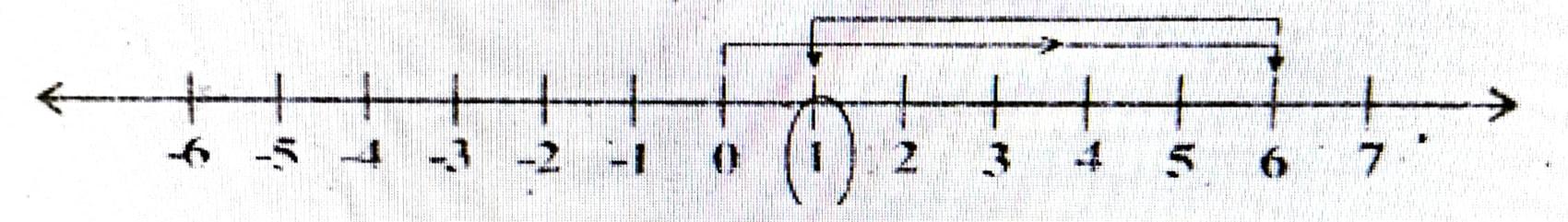
(iv) (-8) + (-3)
Ans: (-8) + (-3)

18. Find whether of the statements are true or false:
(i) (-6) + 23 + (-2) = (-2) + (-6) + 23
Ans: Correct.
(ii) {(16 -15) + (-7) = 16 – {15 + (-7)}
Ans: Correct.
(iii) Natural numbers are closed under subtraction.
Ans: Incorrect.
(iv) Of the two numbers 0 and -670, -670 is greater.
Ans: Incorrect.
(v) With respect to subtraction of integers commutative property and associative property do not hold.
Ans: Correct.
Exercise – 1.2 |
|---|
1. Find the product
(i) 5 × (- 2)
Ans: 5 × (2)
= -10
(ii) (-3) × 7
Ans: (-3) × 7
= -21
(iii) (-4) × (-3)
Ans: (-4) × (-3)
= 4 × 3
= 12
(iv) (-129) × (-1)
Ans: (-129) × (-1)
= (129 × 1)
= 129
(v) (-12) × 0 × (-17)
Ans: (-12) × 0 × (-17)
= (12 × 17) × 0
= 0
(vi) (-22) × (-11) × 10
Ans: (-22) × (-11) × 10
= (22 × 11) × 10
= 242 × 10
= 2420
(vii) 13 × (-5) × (-3)
Ans: 13 × (-5) × (-3)
= 13 × 5 × 3
= 195
(viii) (-27) × (-31) × (-2)
Ans: (-27) × (-31) × (-2)
= -(27 × 31 × 2)
= -1674
(ix) (-3) × (-1) × (-2) × 5
Ans: (-3) × (-1) × (-2) × 5
= -(3 × 1 × 2 × 5)
= -30
2. Verify whether true or false
(i) 27 × {(-5) + 10} = 27 × (-5) + 27 × 10
Ans: L.H.S. = 27 × {(-5) + 10}
= 27 × 5
= 135
R.H.S. = 27 × (-5) + 27 × 10
= – 135 + 270
= 135
L.H.S. = R.H.S. True.
(ii) (-25) × {(-16) + (-24)} = (-25) × (-16) × (-24)
Ans: L.H.S. = (-25) × {(-16 – 24) = { – (25 × 16 × 24)}
⇒ (25) × (-40) = -(9600)
∴ L.H.S ≠ R.H.S.
(iii) a – b = a + b, where a = (-75), b = (-20)
Ans: L.H.S. = a – (-b)
= a + b
= (75) + (-20)
= -75 – 20 = -(75 + 20) = -95
R.H.S. = a + b = (-75) + (-20)
= -(75 + 20) = -95
∴ L.H.S. = R.H.S.
3. (i) Product of any two integers is -33. If one of them is 11, what is the other number?
Ans: Product of any two integers is = -33 and one of them is = 11.
∴ Other number = – 33/11 = -3.
(ii) Product of any two integers is 51. If one of them is -1, what is the other number?
Ans: Product of any two integers is = 51 and one of them is 1.
∴ other number = 51/-1 = – 51
(iii) What is the value of (-1 × a) for any integer ‘a’?
Ans: The value of (-1 × a) for any integer ‘a’ = -a.
4. Find the product applying appropriate property-
(i) 125 × (-54) × 8
Ans: 125 × (-54) × 8
= {125 × 8} × (-54) [Commutative Property]
= 1000 × (-54)
= -54000
(ii) (-25) × (-97) × 4
Ans: (-25) × (-97) × 4
= {(- 25) ×4} × (-97) [Commutative Property]
= (-100) × (-97)
= 9700
(iii) (-27) × (-33)
Ans: (-27) × (-33)
= -30 × (-30 + 3)
= (-33) × (-30) + 3 × (-33) [Associative Property]
= 990 – 99 [Distributive Property]
= 891
(iv) 25 × (-58) + (-58) × (-35)
Ans: 25 × (-58) + (-58) × (-35)
= -58{25 + (-35)} [Distributive Property]
= -58(25 – 35)
= -58 × (-10)
= 580
(v) 15 × (-25) × (-4) × (-10)
Ans: 15 × (-25) × (-4) × (-10)
= {(-25) × (-4)} × {15 × (-10)} [Commutative Property]
= 100 × (-150)
= -15000
(vi) (-57) × (-19) × 57
Ans: (-57) × (-19) × 57
= (-57) × 57 × (-19) [Commutative Property]
= (-3249) × (-19)
= 61,731
5. Evaluate with the help of distributive and associative property:
(i) 125 × (54) × 8
Ans: 125 × (54) × 8
= {125 × 8} × 54 [Commutative Property]
= (100 + 25) × 8 × 54 [Associative Property]
= (800 + 200) × 54
= 1000 × 54
= 54,000
(ii) (-25) × 75 × 8 × (-4)
Ans: (-25) × 75 × 8 × (-4)
= (-25) × (-4) × 75 × 8 [Commutative Property]
= 100 × 600
= 60,000
(iii) 225 × 67 × 3
Ans: 225 × 67 × 3
= (225 × 3) × 67 [Commutative Property]
= 225 × 3 × (70 – 3) [Associative Property]
= 675 × (70 – 3)
= 675 × 70 – 675 × 3
= 47250 – 2025 = 45,225
6. Evaluate using distributive property:
(i) 172 × 25 + 172 × 35
Ans: 172 × 25 + 172 × 35
= 172 × (25 + 35) [Distributive Property]
= 172 × 60
= 10320
(ii) 159 × 82 + 159 × 16 + 159 × 2
Ans: 159 × 82 + 159 × 16 + 159 × 2
= 159 × (82 + 16 + 2) [Distributive Property]
= 159 × 100
= 15900
(iii) 67 × 78 + 67 × (-43) + 67 × (-25)
Ans: 67 × 78 + 67 × (-43) + 67 × (-25)
= 67 × (78 – 43 – 25) [Distributive Property]
= 67(78 – 43 – 25)
= 67 × (78 – 68)
= 67 × 10
= 670
(iv) 999 × 99 + 99
Ans: 999 × 99 + 99
= 99 × (999 + 1) [Distributive Property]
= 99 × 1000
= 99000
(v) 58 × 47 + 94
Ans: 58 × 47 + 94
= 58 × 47 + 47 × 2 [Distributive Property]
= 47(58 + 2)
= 47 × 60
= 2,820
7. Justify whether right or wrong:
(i) (-7) × 15 × (-4) = (-7) × 15 + (-7) × (-4)
Ans: (-7) × 15 × (-4) = (-7) × 15 + (-7) × (-4)
⇒ 28 × 15 = (-7){15 + (-4)}
⇒ 28 × (20 – 5) = (-7) × (15 – 4)
⇒ 28 × 20 – 28 × 5 = (-7) × (15 – 4)
⇒ 560 – 140 = (-7) × 11
⇒ 420 = -77
∴ Incorrect.
(ii) (-6) × 23 × (-2) = (-2) × (-6) × 23
Ans: (-6) × 23 × (-2) = (-2) × (-6) × 23
= (-6) × 23 × (-2), Correct.
(iii) (-5) × {(-3) × 2) = {(-5) × (-3)} x 2
Ans: (-5) × {(-3) × 2) = {(-5) × (-3)} × 2
= (-5) × {(-3) × 2}, Correct.
(iv) (-175) × (-1) = -175
Ans: (-175) × (-1) = -175
⇒ 175 = -175 Incorrect.
(v) (-25) × (-4) × 0 = 100
Ans: (-25) × (-4) × 0 = 100
⇒ 100 × 0 = 100
⇒ 0 = 100 Incorrect.
Exercise – 1.3 |
|---|
1. Find the quotient
(i) 14 ÷ (-5)
Ans: 14 ÷ (-5)
= 14/-5
= -2.8
(ii) (-60) ÷ 10
Ans: (-60) ÷ 10
= -60/10
= -6
(iii) (-54) ÷ (-6)
Ans: (-54) ÷ (-6)
= -54/-6
= 9
(iv) 0 ÷ (-15)
Ans: 0 ÷ (-15)
= 0/-15
= 0
(v) (-61) ÷ {(-60) + (-1)}
Ans: (-61) ÷ {(-60) + (-1)}
= (-61) ÷ (-61 – 1)
= -61/-61
= 1
(vi) [{(-72) ÷ (-6)} ÷ (-3)]
Ans: [{(-72) ÷ (-6)} ÷ (-3)]
= -72/-6 ÷ (-3)
= 12 ÷ (-3)
= -4
2. Fill in the blanks:
(i) (-600) ÷ 25 = ____________
Ans: (-600) ÷ 25 = -24
(ii) {(-4) × 18} ÷ ____________ = 12
Ans: {(-4) × 18} ÷ -6 = 12
(iii) _____________ ÷ (5 – 6) = -20
Ans: 20 ÷ (5 – 6) = -20
(iv) (-123) ÷ (-1) = _____________
উত্তৰঃ (-123) ÷ (-1) = 123
3. (i) If a / (- 7) = 8 then find the value of integer ‘a’.
Ans: a ÷ (-7) = 8
⇒ a/-7 = 8
⇒ a = -56
(ii) If 125 / b = – 5 then find the value of integer ‘b’.
Ans: 125 ÷ b = -5
⇒ 125/b = 5
⇒ (-5) × b = 125
⇒ b = 125/-5 = -25
4. Write three pairs of integers a, b such that a ÷ b = -5.
Ans: Three pairs of integers a, b such that a ÷ b = -5 are (15, -3), (45, -9) and (25, -5).
5. In a class test of a school 20 questions were given. For each correct answer 5 marks is awarded and for each wrong answer (-2) is awarded.
(i) A student answered all the questions. But her 10 answers were correct. How much makes did she score?
Ans: A student answered all the questions, but her 10 answers were correct.
Marks for 10 correct answer = 10 × 5 = 50
and Marks for 10 incorrect answers
= 10(-2)
= -20
∴ She scored = (50 – 20) = 30.
(ii) Other student could answer only 5 answers correctly. What was the score by the student?
Ans: Other student could answer only 5 answers correctly.
∴ Marks scored for 5 correct answers = 5 × 5
= 25 and marks scored for 15 incorrect answer
= 15(-2)
= – 30.
The score by the student = (-30 + 25) = -5 marks.
6. In an examination for each correct answer 5 marks is awareded and for each wrong answer (-2) is awarded.
(i) Sumon answered all the questions. But her 16 answers were correct and obtained 64.
Ans: Sumon answered all the questions, but her 16 answers were correct and obtained 64.
Marks scored for 16 correct answers = 16 × 5 = 80
∴ Marks scored for incorrect answers = (80 – 64) = 16.
For each wrong answer (-2) is awarded
∴ Number of questions answered incorrect = 16/2 = 8
(ii) Jaya answered all the questions. But she could answer only 5 questions correctly and obtained (-6). How many questions were answered incorrectly by them?
Ans: Jaya answered all the questions.
Only 5 questions correctly and obtained (-6).
∴ Marks scored for correct answers = 6 × 5 = 30
For (-6) marks, number of incorrect answers = 3
∴ 30/2 = 15 and 6/2 = 3
∴ Jaya answered incorrectly = (15 + 3) = 18 questions.
7. A rubber company makes a profit of Rs. 15 per bag of rubber. Loss incured on every waste bag of rubber is of Rs. 8.
(i) The Company sold 1500 bags of good rubber and 500 bags of waste rubber. How much profit or loss incurred by the company?
Ans: Profit on per bag of rubber = Rs. 15.00
Profit for 1500 bags of rubber
= Rs. (1500 × 15)
= Rs. 22500
Loss of 500 bags of waste rubber
= Rs. (500 × 8)
= Rs. 4000
∴ Profit Rs. (22, 500 – 4,000)
= Rs. 18,500.
(ii) If the company sold 750 bags of waste rubber then how many bags of good rubber are to be sold so that company incured neither profit nor loss?
Ans: Loss for 750 bags of waste rubber
= Rs. (750 × 8)
= Rs. 6,000
∴ Number of bags of good rubber are to be sold so that company incurred neither profit nor loss
= (6,000 ÷ 15)
= 400.
FAQs
1. What topics are covered in SEBA Class 7 Maths Chapter 1 Integers?
This chapter covers addition, subtraction, multiplication, and division of integers, along with their properties such as associative, commutative, and distributive laws.
2. How many exercises are there in Chapter 1 Integers?
There are three exercises: Exercise 1.1, Exercise 1.2, and Exercise 1.3, each focusing on different operations and properties of integers.
3. Are these solutions available in English Medium?
Yes, all solutions for SEBA Class 7 Maths Chapter 1 are available in English Medium, as per the SCERT Assam syllabus.
4. How do I access SEBA Class 7 Maths Chapter 1 solutions?
You can access all solutions on our website or scan the provided QR code for quick access.
5. Why is learning Integers important in Mathematics?
Integers are fundamental in mathematics, forming the basis for algebra, equations, and real-life applications like temperature changes, banking, and elevations.
Get Free NCERT PDFs
If you want to download free PDFs of any chapter, click the link below and join our WhatsApp group:


